Table of Contents | Introduction | Home
![]()
P l a n e G e o m e t r y
An Adventure in Language and Logic
based on

BOOK I
ADDITIONAL EXERCISES
| 11. | Any point on the perpendicular bisector of a straight line is equidistant from the endpoints of the straight line. |
| 12. | When one straight line stands on another, the bisectors of the adjacent angles are perpendicular to one another. |
| 13. | The straight lines which join the midpoints of the sides of an equilateral triangle form another equilateral triangle. |
| 14. | If a straight line intersects one of two parallel lines, it also intersects the other. |
| 15. | If two straight lines intersect, then any perpendiculars to each line, if extended, will also intersect. |
| 16. | If from any point outside of an acute angle perpendiculars are drawn to each side of the acute angle, then the angle contained by those perpendiculars is equal to the acute angle. |
| 17. | If the equal sides of an isosceles triangle are extended, then the angles formed under the base are equal to one another. |
| 18. | If the angles formed under the base of a triangle are equal to one another, then the triangle is isosceles. |
| 19. | To draw a straight line at right angles to a given straight line but from one end of it, and the given line may not be extended. |
| 10. | If the sides of one angle are parallel to the sides of another angle, then the two angles are either equal or supplementary. |
| 11. | If perpendiculars are drawn from each side of a given angle, then the angle formed by those perpendiculars is equal to the supplement of the given angle. |
| 12. | The diagonals of a parallelogram bisect each other. |
| 13. | The diagonals of a rectangle are equal to one another. |
| 14. | The diagonals of a rhombus are perpendicular to one another. |
| 15. | The straight line that joins the vertex of an isosceles triangle and the midpoint of the base, also cuts the base at right angles. |
| 16. | In a quadrilateral if both pairs of opposite angles are equal, then the figure is a parallelogram. |
| 17. | If the straight line that bisects an exterior angle of a triangle is parallel to a side, then the triangle is isosceles. |
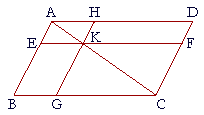
| 18. | In any parallelogram the complements of the parallelograms about the diagonal are equal to one another. (This is Proposition I. 43.) |
 |
|
| Let ABCD be a parallelogram and AC its diagonal; let EH, GF be parallelograms about AC, that is, through which AC passes; and let BK, KD be the other parallelograms that make up the whole figure ABCD, and are therefore called the complements: then the complement BK is equal (in area) to the complement KD. |
|
| * | * | * |
Table of Contents | Introduction | Home
Copyright © 2021 Lawrence Spector
Questions or comments?
E-mail: teacher@themathpage.com